Moving sofa problem

In mathematics, the moving sofa problem or sofa problem is a two-dimensional idealisation of real-life furniture-moving problems and asks for the rigid two-dimensional shape of largest area that can be maneuvered through an L-shaped planar region with legs of unit width.[1] The area thus obtained is referred to as the sofa constant. The exact value of the sofa constant is an open problem. The currently leading solution, by Joseph L. Gerver, has a value of approximately 2.2195 and is thought to be close to the optimal, based upon subsequent study and theoretical bounds.

Unsolved problem in mathematics:

What is the largest area of a shape that can be maneuvered through a unit-width L-shaped corridor?

(more unsolved problems in mathematics)

History

The first formal publication was by the Austrian-Canadian mathematician Leo Moser in 1966, although there had been many informal mentions before that date.[1]

Bounds

Work has been done on proving that the sofa constant (A) cannot be below or above certain values (lower bounds and upper bounds).

Lower

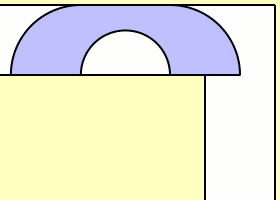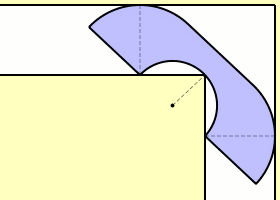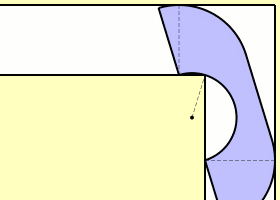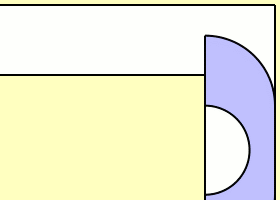
The Hammersley sofa has area 2.2074 but is not the largest solution

Gerver's sofa of area 2.2195 with 18 curve sections

An obvious lower bound is $A\geq \pi /2\approx 1.57$ . This comes from a sofa that is a half-disk of unit radius, which can rotate in the corner.

John Hammersley derived a lower bound of $A\geq \pi /2+2/\pi \approx 2.2074$ based on a shape resembling a telephone handset, consisting of two quarter-disks of radius 1 on either side of a 1 by $4/\pi$ rectangle from which a half-disk of radius $2/\pi$ has been removed.[2][3]

In 1992, Joseph L. Gerver of Rutgers University described a sofa described by 18 curve sections each taking a smooth analytic form. This further increased the lower bound for the sofa constant to approximately 2.2195.[4][5]

Upper

Hammersley also found an upper bound on the sofa constant, showing that it is at most $2{\sqrt {2}}\approx 2.8284$ .[1][6]

Yoav Kallus and Dan Romik proved a new upper bound in June 2017, capping the sofa constant at $2.37$ .[7]

Ambidextrous sofa

Romik's ambidextrous sofa

A variant of the sofa problem asks the shape of largest area that can go round both left and right 90 degree corners in a corridor of unit width (where the left and right corners are spaced sufficiently far apart that one is fully negotiated before the other is encountered). A lower bound of area approximately 1.64495521 has been described by Dan Romik. His sofa is also described by 18 curve sections.[8][9]

References

Wagner, Neal R. (1976). "The Sofa Problem" (PDF). The American Mathematical Monthly. 83 (3): 188–189. doi:10.2307/2977022. JSTOR 2977022. Archived from the original (PDF) on 2015-04-20. Retrieved 2009-07-25.
Croft, Hallard T.; Falconer, Kenneth J.; Guy, Richard K. (1994). Halmos, Paul R. (ed.). Unsolved Problems in Geometry. Problem Books in Mathematics; Unsolved Problems in Intuitive Mathematics. Vol. II. Springer-Verlag. ISBN 978-0-387-97506-1. Retrieved 24 April 2013.
Finch, Steven, Moving Sofa Constant, Mathcad Library (includes a diagram of Gerver's sofa).
Gerver, Joseph L. (1992). "On Moving a Sofa Around a Corner". Geometriae Dedicata. 42 (3): 267–283. doi:10.1007/BF02414066. ISSN 0046-5755. S2CID 119520847.
Weisstein, Eric W. "Moving sofa problem". MathWorld.
Stewart, Ian (January 2004). Another Fine Math You've Got Me Into... Mineola, N.Y.: Dover Publications. ISBN 0486431819. Retrieved 24 April 2013.
Kallus, Yoav; Romik, Dan (December 2018). "Improved upper bounds in the moving sofa problem". Advances in Mathematics. 340: 960–982. arXiv:1706.06630. doi:10.1016/j.aim.2018.10.022. ISSN 0001-8708. S2CID 5844665.
Romik, Dan (2017). "Differential equations and exact solutions in the moving sofa problem". Experimental Mathematics. 26 (2): 316–330. arXiv:1606.08111. doi:10.1080/10586458.2016.1270858. S2CID 15169264.
Romik, Dan. "The moving sofa problem - Dan Romik's home page". UCDavis. Retrieved 26 March 2017.

External links

Romik, Dan (March 23, 2017). "The Moving Sofa Problem" (video). YouTube. Brady Haran. Archived from the original on 2021-12-21. Retrieved 24 March 2017.
SofaBounds - Program to calculate bounds on the sofa moving problem.
A 3D model of Romik's ambidextrous sofa

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

[Neal_Wagner-1] Wagner, Neal R. (1976). "The Sofa Problem" (PDF). The American Mathematical Monthly. 83 (3): 188–189. doi:10.2307/2977022. JSTOR 2977022. Archived from the original (PDF) on 2015-04-20. Retrieved 2009-07-25.

[2] Croft, Hallard T.; Falconer, Kenneth J.; Guy, Richard K. (1994). Halmos, Paul R. (ed.). Unsolved Problems in Geometry. Problem Books in Mathematics; Unsolved Problems in Intuitive Mathematics. Vol. II. Springer-Verlag. ISBN 978-0-387-97506-1. Retrieved 24 April 2013.

[3] Finch, Steven, Moving Sofa Constant, Mathcad Library (includes a diagram of Gerver's sofa).

[4] Gerver, Joseph L. (1992). "On Moving a Sofa Around a Corner". Geometriae Dedicata. 42 (3): 267–283. doi:10.1007/BF02414066. ISSN 0046-5755. S2CID 119520847.

[5] Weisstein, Eric W. "Moving sofa problem". MathWorld.

[6] Stewart, Ian (January 2004). Another Fine Math You've Got Me Into... Mineola, N.Y.: Dover Publications. ISBN 0486431819. Retrieved 24 April 2013.

[7] Kallus, Yoav; Romik, Dan (December 2018). "Improved upper bounds in the moving sofa problem". Advances in Mathematics. 340: 960–982. arXiv:1706.06630. doi:10.1016/j.aim.2018.10.022. ISSN 0001-8708. S2CID 5844665.

[Dan_Romik-8] Romik, Dan (2017). "Differential equations and exact solutions in the moving sofa problem". Experimental Mathematics. 26 (2): 316–330. arXiv:1606.08111. doi:10.1080/10586458.2016.1270858. S2CID 15169264.

[UCDavis-9] Romik, Dan. "The moving sofa problem - Dan Romik's home page". UCDavis. Retrieved 26 March 2017.