Pearcey integral

In mathematics, the Pearcey integral is defined as[1]

${\displaystyle \operatorname {Pe} (x,y)=\int _{-\infty }^{\infty }\exp(i(t^{4}+xt^{2}+yt))\,dt.}$

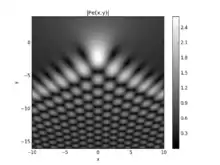
A plot of the absolute value of the Pearcey integral as a function of its two parameters.

A photograph of a cusp caustic produced by illuminating a flat surface with a laser beam through a droplet of water.

The Pearcey integral is a class of canonical diffraction integrals, often used in wave propagation and optical diffraction problems[2] The first numerical evaluation of this integral was evaluated using the quadrature formula in Trevor Pearcey.[3][4]

In optics, the Pearcey integral can be used to model diffraction effects at a cusp caustic.